by Mr Johari
source: http://www.mathsisfun.com/algebra/quadratic-equation-real-world.html
REAL LIFE APPLICATION OF QUADRATIC EQUATIONEXAMPLE 1
 | Balls, Arrows, Missiles and Stones
If you throw a ball (or shoot an arrow, fire a missile or throw a stone) it will go up into the air, slowing down as it goes, then come down again ...
... and a Quadratic Equation tells you where it will be!
Example: Throwing a BallA ball is thrown straight up, from 3 m above the ground, with a velocity of 14 m/s. When does it hit the ground? |
Ignoring air resistance, we can work out its height by adding up these three things:
| The height starts at 3 m: | 3 | |
| It travels upwards at 14 meters per second (14 m/s): | 14t | |
| Gravity pulls it down, changing its speed by about 5 m/s per second (5 m/s2): | -5t2 | |
| (Note for the enthusiastic: the -5t2 comes from -½at2 with a=9.81 m/s2) |
Add them up and the height h at any time t is:
h = 3 + 14t - 5t2
And the ball will hit the ground when the height is zero:
3 + 14t - 5t2 = 0
Which is a Quadratic Equation ! In "Standard Form" it looks like:
-5t2 + 14t + 3 = 0
Let us solve it ...
There are many ways to solve it, here we will use the factoring method:
| Will be easier if we multiply all terms by -1: | 5t2 - 14t - 3 = 0 | |
Now our job is to factor it. We will use the "Find two numbers that
multiply to give a×c, and add to give b" method in Factoring Quadratics.
a×c = -15, and b = -14.
The positive factors of -15 are 1, 3, 5, 15, and one of the
factors has to be negative. By trying a few we find that -15 and 1 work (-15×1=-15, and -15+1= -14) | ||
| Rewrite middle with -15 and 1: | 5t2 - 15t + t - 3= 0 | |
| Factor first two and last two: | 5t(t - 3) + 1(t - 3) = 0 | |
| Common Factor is (t - 3): | (5t + 1)(t - 3) = 0 | |
| And the two solutions are: | 5t + 1 = 0 or t - 3 = 0 | |
| t = -0.2 or t = 3 | ||
The "t = -0.2" is a negative time, impossible in our case.
The "t = 3" is the answer we want:
The ball hits the ground after 3 seconds!
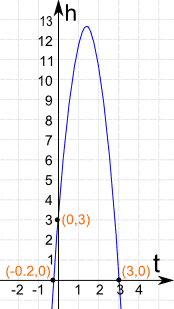 |
Here is the graph of the Parabola h = -5t2 + 14t + 3
It shows you the height of the ball vs time
Some interesting points:
(0,3) When t=0 (at the start) the ball is at 3 m
(-0.2,0) Says that -0.2 seconds BEFORE we threw the ball it was at ground level ... this never happened, so our common sense says to ignore it!
(3,0) Says that at 3 seconds the ball is at ground level.
Note also that the ball reaches nearly 13 meters high.
|
Note for the enthusiastic: You can find exactly where the top point is! The method is explained in Graphing Quadratic Equations, and has two steps:
Find where (along the horizontal axis) the top occurs using -b/2a:
- t = -b/2a = -(-14)/(2 × 5) = 14/10 = 1.4 seconds
Then find the height using that value (1.4)
- h = -5t2 + 14t + 3 = -5(1.4)2 + 14 × 1.4 + 3 = 12.8 meters
So the ball reaches the highest point of 12.8 meters after 1.4 seconds.
===========================================================
EXAMPLE 2
Example: New Sports Bike
 | You have designed a new style of sports bicycle!
Now you want to make lots of them and sell them for profit.
|
Your costs are going to be:
- $700,000 for manufacturing set-up costs, advertising, etc
- $110 to make each bike
Based on similar bikes, you can expect sales to follow this "Demand Curve":
Where "P" is the price.
For example, if you set the price:
| 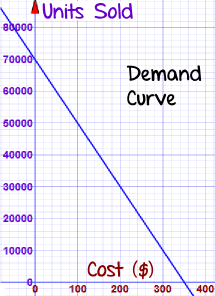 |
So ... what is the best price? And how many should you make?
Let us make some equations!
How many you sell depends on price, so use "P" for Price as the variable
- Unit Sales = 70,000 - 200P
- Sales in Dollars = Units × Price = (70,000 - 200P) × P = 70,000P - 200P2
- Costs = 700,000 + 110 x (70,000 - 200P) = 700,000 + 7,700,000 - 22,000P = 8,400,000 - 22,000P
- Profit = Sales-Costs = 70,000P - 200P2 - (8,400,000 - 22,000P) = -200P2 + 92,000P - 8,400,000
Profit = -200P2 + 92,000P - 8,400,000
Yes, a Quadratic Equation. Let us solve this one by Completing the Square.
Solve: -200P2 + 92,000P - 8,400,000 = 0
Step 1 Divide all terms by -200
P2 – 460P + 42000 = 0
Step 2 Move the number term to the right side of the equation:
P2 – 460P = -42000
Step 3 Complete the square on the left side of the equation and balance this by adding the same number to the right side of the equation:
(b/2)2 = (-460/2)2 = (-230)2 = 52900
P2 – 460P + 52900 = -42000 + 52900
(P – 230)2 = 10900
Step 4 Take the square root on both sides of the equation:
P – 230 = ±√10900 = ±104 (to nearest whole number)
Step 5 Subtract (-230) from both sides (in other words, add 230):
P = 230 ± 104 = 126 or 334
What does that tell us? It says that the profit will be ZERO when the Price is $126 or $334
But we want to know the maximum profit, don't we?
It will be exactly half way in-between! At $230
And here is the graph:
Profit = -200P2 + 92,000P - 8,400,000
The optimum sale price is $230, and you can expect:
| 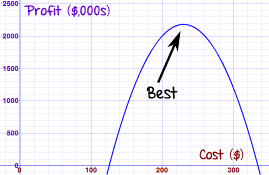 |
A very profitable venture.
===========================================================
EXAMPLE 3
Example: Small Steel Frame
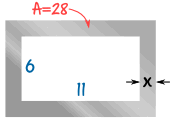 |
Your company is going to make frames as part of a new product they are launching.
The frame will be cut out of a piece of steel, and to keep the weight down, the final area should be 28 cm2
The inside of the frame has to be 11 cm by 6 cm
What should the width x of the metal be?
|
Area of steel before cutting:
Area = (11 + 2x) × (6 + 2x) cm2
Area = 66 + 22x + 12x + 4x2
Area = 4x2 + 34x + 66
Area of steel after cutting out the 11 × 6 middle:
Area = 4x2 + 34x + 66 - 66
Area = 4x2 + 34x
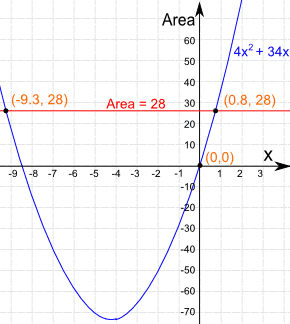
Let us solve this one graphically!
Here is the graph of 4x2 + 34x :
The required area of 28 is shown as a horizontal line.
The area equals 28 cm2 when:
x is approximately -9.3 or 0.8
The negative value of x make no sense, so the answer is:
x = 0.8 cm (approx.)
|  |
===========================================================
EXAMPLE 4
Example: River Cruise
A 3 hour river cruise goes 15 km upstream and then back again. The river has a current of 2 km an hour. What is the boat's speed and how long was the upstream journey?
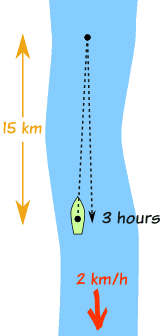 |
There are two speeds to think about: the speed the boat makes in the water, and the speed relative to the land:
Because the river flows downstream at 2 km/h:
We can turn those speeds into times using:
time = distance / speed
(if you travel 8 km at 4 km/h it would take 8/4 = 2 hours, right?)
|
And we know the total time is 3 hours:
total time = time upstream + time downstream = 3 hours
Put all that together:
total time = 15/(x-2) + 15/(x+2) = 3 hours
Now we use our algebra skills to solve for "x".
First, get rid of the fractions by multiplying through by (x-2)(x+2):
3(x-2)(x+2) = 15(x+2) + 15(x-2)
Expand everything:
3(x2-4) = 15x+30 + 15x-30
Bring everything to the left and simplify:
3x2 - 30x - 12 = 0
It is a Quadratic Equation! Let us solve it using the Quadratic Formula:
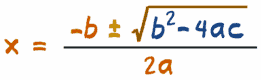
Where a, b and c are from the
Quadratic Equation in "Standard Form": ax2 + bx + c = 0
Quadratic Equation in "Standard Form": ax2 + bx + c = 0
Solve 3x2 - 30x - 12 = 0
| Coefficients are: | a = 3, b = -30 and c = -12 | |
| Quadratic Formula: | x = [ -b ± √(b2-4ac) ] / 2a | |
| Put in a, b and c: | x = [ -(-30) ± √((-30)2-4×3×(-12)) ] / (2×3) | |
| Solve: | x = [ 30 ± √(900+144) ] / 6 | |
| x = [ 30 ± √(1044) ] / 6 | ||
| x = ( 30 ± 32.31 ) / 6 | ||
| x = -0.39 or 10.39 |
Answer: x = -0.39 or 10.39 (to 2 decimal places)
x =-0.39 makes no sense for this real world problem, but x = 10.39 is just perfect!
Answer: Boat's Speed = 10.39 km/h (to 2 decimal places)
And hence the upstream journey = 15 / (10.39-2) = 1.79 hours = 1 hour 47min
And the downstream journey = 15 / (10.39+2) = 1.21 hours = 1 hour 13min
No comments:
Post a Comment